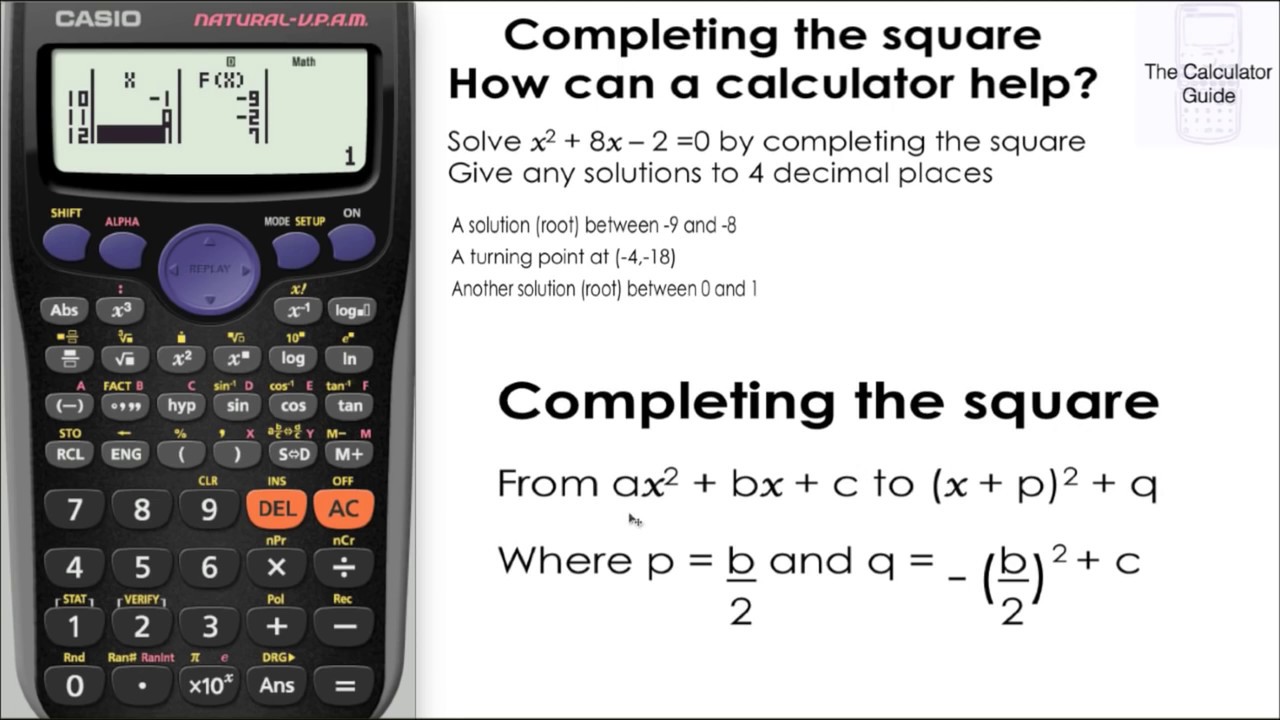
Completing the square is a fundamental technique in algebra that transforms quadratic equations into a more manageable format. This method not only aids in solving equations but also plays a crucial role in graphing parabolas and understanding their properties. With the advent of technology, tools like the completing the square calculator have made this process simpler and more efficient, enabling both students and professionals to tackle complex problems with ease.
In a world where mathematical accuracy is paramount, having a reliable completing the square calculator at your fingertips can significantly enhance your problem-solving capabilities. This calculator offers instant solutions to quadratic equations by converting them into vertex form, making it easier to analyze and interpret the results. Whether you’re a student grappling with homework or a professional in need of quick calculations, this tool is indispensable.
The beauty of completing the square lies not only in its utility but also in its underlying principles. By breaking down a quadratic equation into a perfect square trinomial, you unlock a deeper understanding of its structure and characteristics. The completing the square calculator simplifies this process, allowing users to focus on the concepts rather than get bogged down by calculations. In this article, we’ll explore the intricacies of completing the square, the advantages of using a calculator, and the steps to effectively utilize this method.
What is Completing the Square?
Completing the square is a method used to rewrite quadratic equations in a specific format. The general form of a quadratic equation is:
Ax² + Bx + C = 0
In order to complete the square, we aim to express this equation in the form:
A(x - h)² + k = 0
Where (h, k) represents the vertex of the parabola. This method not only helps in finding the roots of the equation but also facilitates graphing the quadratic function.
Why Use a Completing the Square Calculator?
The completing the square calculator is a valuable tool for several reasons:
- Time-Saving: It provides instant solutions without the need for lengthy calculations.
- Accuracy: Reduces the risk of human error in calculations.
- Ease of Use: User-friendly interfaces make it accessible for all levels of users.
- Educational Value: It can help users understand the process and logic behind completing the square.
How Does a Completing the Square Calculator Work?
A completing the square calculator typically follows these steps:
- Input the coefficients A, B, and C of the quadratic equation.
- The calculator rearranges the equation to isolate the constant term.
- It adds and subtracts the necessary value to form a perfect square trinomial.
- Finally, it outputs the equation in vertex form and provides the vertex coordinates.
Can Completing the Square Help in Graphing?
Yes, completing the square is an essential technique for graphing quadratic functions. By converting the equation into vertex form, you can easily identify the vertex of the parabola, which is a crucial point in graphing.
What are the Steps to Complete the Square Manually?
To complete the square manually, follow these steps:
- Start with the quadratic equation in standard form.
- If A is not equal to 1, divide the entire equation by A.
- Move the constant term to the other side of the equation.
- Add the square of half the coefficient of x to both sides.
- Factor the left-hand side and simplify the right-hand side.
What are the Benefits of Learning to Complete the Square?
Understanding how to complete the square offers several advantages:
- Stronger Mathematical Foundation: It deepens your comprehension of quadratic functions.
- Enhanced Problem-Solving Skills: It equips you with a versatile tool for various types of mathematical problems.
- Improved Graphing Abilities: It enables you to accurately graph parabolas and identify key features.
Are There Limitations to Using a Completing the Square Calculator?
While a completing the square calculator is highly effective, it does have some limitations:
- Dependency on Technology: Over-reliance may hinder the development of manual calculation skills.
- Understanding the Process: Users may miss out on the learning experience if they skip manual steps.
- Specificity: Some calculators may not handle complex or non-standard equations.
Conclusion: Embrace the Power of Completing the Square
The completing the square calculator is a powerful ally in your mathematical journey. By simplifying the process and providing instant solutions, it allows you to focus on understanding the concepts rather than getting lost in calculations. Whether you're a student, educator, or professional, mastering the art of completing the square will undoubtedly enhance your mathematical prowess. So, embrace this technique and let the completing the square calculator guide you through the world of quadratics!